LaTeX templates and examples — Assignments
Handy LaTeX templates for homework assignments to use at school, college, and university. They’re great for teachers to prep classes too.
Recent
Homework Template for UWYO COSC-4765 Computer Security - Homework #2
standard template for a uni assessment
Template for CS 109 PSET at Stanford
Homework template for MATH 304 Spring 2017
Az egész együtthatós polinomok Q és Z feletti felbontásainak kapcsolatáról szóló tétel bizonyítása. (Az SZTE matematika alapszak Algebra és számelmélet (MBNK13) kurzusához házi feladat.)
A test feletti polinomok maradékos osztásáról szóló tétel bizonyítása. (Az SZTE matematika alapszak Algebra és számelmélet (MBNK13) kurzusához házi feladat.)
One of the acceptable templates for writing a physics comps paper at Carleton College. This template is part of an internal wiki page for students at Carleton College.
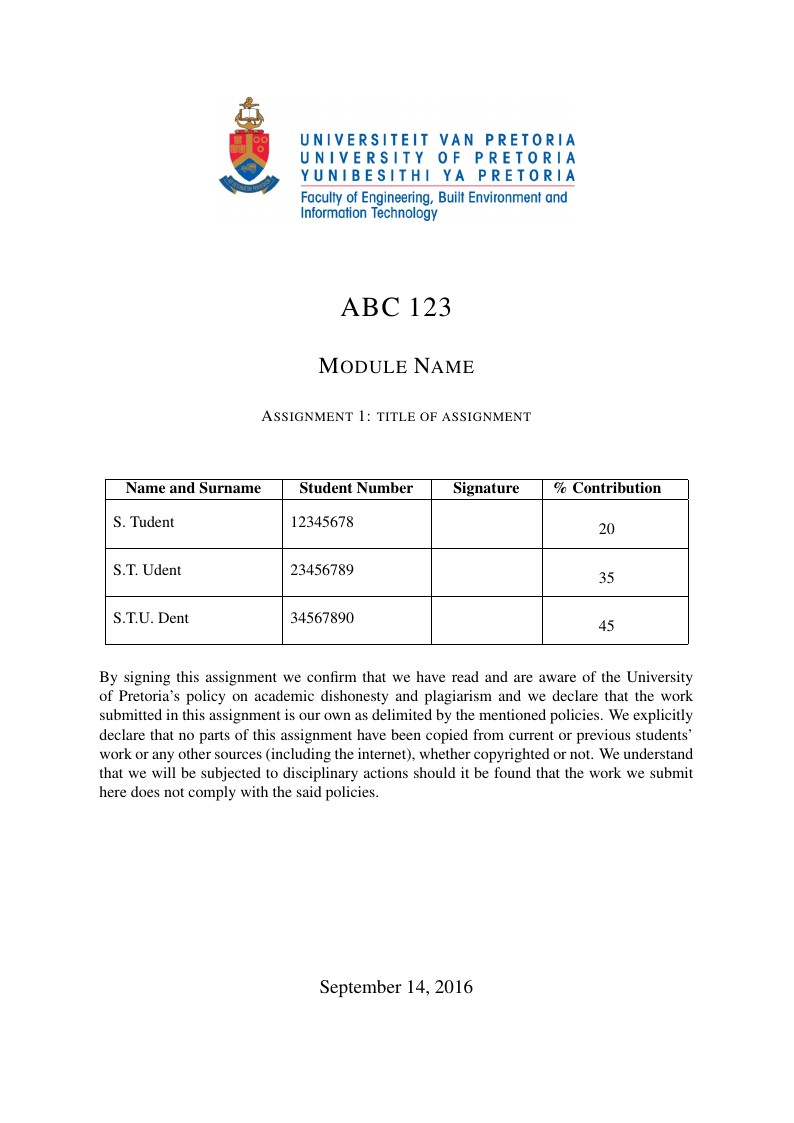
LaTeX template for EECE Undergraduates at University of Pretoria
This is the template for DAM (discrete and argumentative mathematics). We prove theorem $2.1$ using the method of proof by way of contradiction. This theorem states that for any set $A$, that in fact the empty set is a subset of $A$, that is $\emptyset \subset A$.
\begin
Discover why over 20 million people worldwide trust Overleaf with their work.